
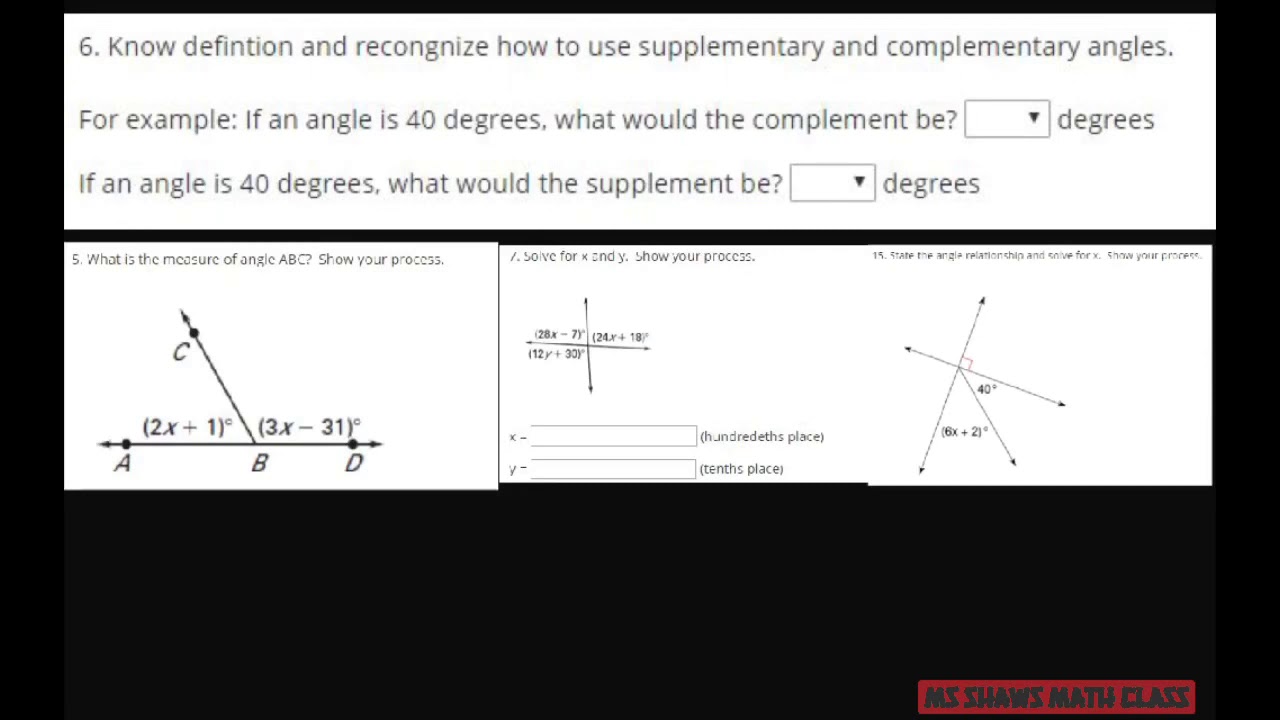

The " exterior " or " external bisector " is the line that divides the supplementary angle ( of 180� minus the original angle ), formed by one side forming the original angle and the extension of the other side, into two equal angles.When a wave train strikes a wall at an oblique angle, the reflected wave train departs at the supplementary angle causing a cross-hatched wave vortices, can erode material from the seabed and transport it along the wall, undermining the structure until it fails.They dont have to be next to each other, just so long as the total is. These are the internal angle bisectors at two opposite vertex angles, the external angle bisectors ( supplementary angle bisectors ) at the other two vertex angles, and the external angle bisectors at the angles formed where the extensions of opposite sides intersect. Supplementary Angles Two Angles are Supplementary when they add up to 180 degrees.Since the sine of an angle and the sine of its supplementary angle are the same, any angle of rotation that maps one of the lines into the other leads to the same value for the spread between the lines.Since the sine of an angle and the sine of its supplementary angle are the same any angle of rotation that maps one of the lines into the other leads to the same value of the spread between the lines.The base angles of an isosceles trapezoid are equal in measure ( there are in fact two pairs of equal base angles, where one base angle is the supplementary angle of a base angle at the other base ).It is not necessary for complementary and supplementary angles to be adjacent, share a vertex and a side, or be next to each other, but they can also be adjacent. Supplementary angles are formed when a ray shares the same vertex and is pointed in a direction that is in between the two original rays that form the straight angle ( 180 degree angle ). It can be said that complementary angles are two angles whose sum is 90 degrees, while supplementary angles are two angles whose sum is 180 degrees.For example, two lines can intersect in no more than one point, intersecting lines have equal opposite angles, and adjacent angles of intersecting lines are supplementary angles.These angles commonly show up in geometry proofs, so if you’re not sure, look for a straight line intersected by another line segment with the two angles sharing a common side and vertex. To recap, adjacent supplementary angles don’t just share a side and vertex but they also add up to 180 degrees. If one vector is pointing the wrong way at a point, you will get the supplementary angle at that point. Recognizing Adjacent Supplementary Angles.When the lines are congruent and several supplementary angles.


 0 kommentar(er)
0 kommentar(er)
